题目描述

小明冒充 𝑋 星球的骑士，进入了一个奇怪的城堡。

城堡里边什么都没有，只有方形石头铺成的地面。

假设城堡地面是 𝑛×𝑛 个方格，如下图所示。

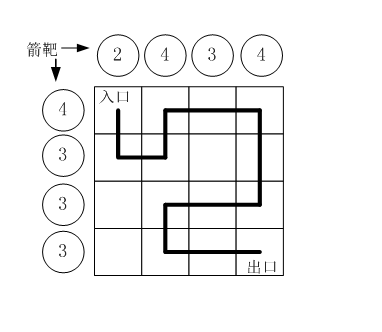
按习俗，骑士要从西北角走到东南角。

可以横向或纵向移动，但不能斜着走，也不能跳跃。

每走到一个新方格，就要向正北方和正西方各射一箭。（城堡的西墙和北墙内各有 𝑛 个靶子）

同一个方格只允许经过一次。

但不必走完所有的方格。

如果只给出靶子上箭的数目，你能推断出骑士的行走路线吗？

有时是可以的，比如上图中的例子。

本题的要求就是已知箭靶数字，求骑士的行走路径（测试数据保证路径唯一）。

#include <iostream>
#include <numeric>
#include<vector>
using namespace std;
typedef long long LL;
int n, num, col[29], row[25], vis[25][25];
int dx[4] = {-1, 0, 1, 0}, dy[4] = {0, 1, 0, -1};

vector<int> path;
bool dfs(int x, int y)    //坐标  
{
    if (vis[x][y] == 1 || x < 0 || y < 0 || x >= n || y >= n) return false; //判断越界;
    if (row[x] == 0 || col[y] == 0) return false;    //不符合题意或找到答案了就返回;
    if (row[x] == 1 && accumulate(row, row + x, 0) != 0)return false;//剪枝
    if (col[y] == 1 && accumulate(col, col + y, 0) != 0)return false;
    row[x]--;
    col[y]--;
    vis[x][y] = 1;
    path.push_back(x * n + y);
    if (x == n - 1 && y == n - 1 && accumulate(col, col + n, 0) == 0 && accumulate(row, row + n, 0) == 0) {    //找到答案就返回;
        return true;
    }
    for (int i = 0; i < 4; i++) {    //右左下上;
        int xx = x + dx[i];
        int yy = y + dy[i];
        if (dfs(xx, yy)) return true;

    }
    col[y]++;
    row[x]++;
    vis[x][y] = 0;
    path.pop_back();
    return false;
}
int main()
{
    cin >> n;
    for (int i = 0; i < n; i++)
    {
        cin >> col[i];
    }
    for (int i = 0; i < n; i++)
    {
        cin >> row[i];
    }

    dfs(0, 0);
    for (int i = 0; i < path.size(); i++)   //从头遍历一遍，输出编号;
        cout << path[i] << " ";
    return 0;
}